A Plus Topper

Improve your Grades

Solving A Quadratic Equation By Completing The Square

by

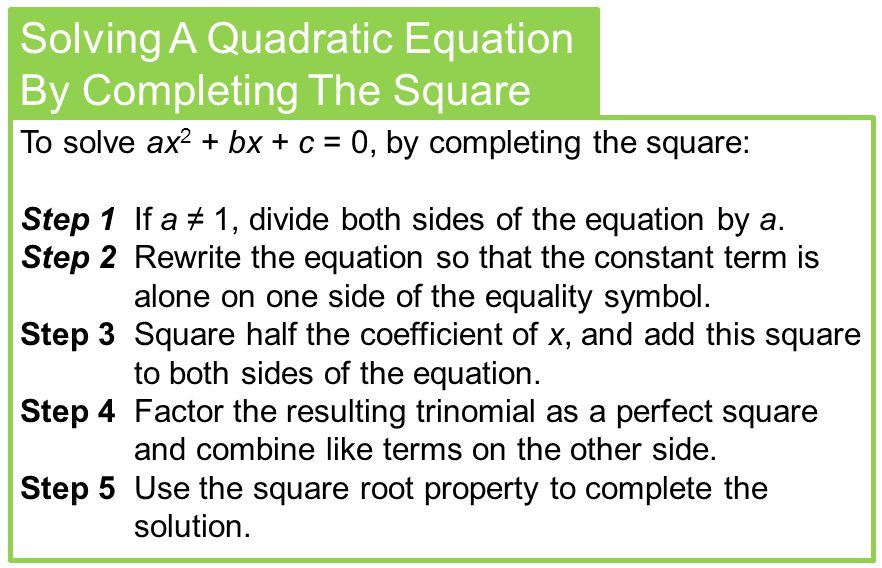
Solving A Quadratic Equation By Completing The Square

Every quadratic equation can be converted in the form:
(x + a)2 – b2 = 0 or (x – a)2 – b2 = 0.
Steps:
1. Bring, if required, all the term of the quadratic equation to the left hand side.
2. Express the terms containing x as x2 + 2xy   or   x2 – 2xy.
3. Add and subtract y2 to get x2 + 2xy + y2 – y2   or   x2 – 2xy + y2 – y2; which gives
(x + y)2 – y2 or (x – y)2 – y2.
Thus,
(i) x2 + 8x = 0 ⇒  x2 + 2x × 4 = 0
⇒ x2 + 2x × 4 + 42 – 42 = 0
⇒ (x + 4)2 – 16 = 0
(ii) x2 – 8x = 0 ⇒ x2 – 2 × x × 4 = 0
⇒ x2 – 2 × x × 4 + 42 – 42 = 0
⇒ (x – 4)2 – 16 = 0

Solving A Quadratic Equation By Completing The Square 1

Solving A Quadratic Equation By Completing The Square With Examples

Example 1:    Find the roots of the quadratic equation 2x2 – 7x + 3 = 0
(if they exist) by the method of completing the square.
Sol.     2x2 – 7x + 3 = 0
\(\Rightarrow {{x}^{2}}-\frac{7}{2}x+\frac{3}{2}=0 \)    [Dividing each term by 2]
\(\Rightarrow {{x}^{2}}-2\times x\times \frac{7}{4}+\frac{3}{2}=0 \)
\(\Rightarrow {{x}^{2}}-2\times x\times \frac{7}{4}+{{\left( \frac{7}{4} \right)}^{2}}-{{\left( \frac{7}{4} \right)}^{2}}+\frac{3}{2}=0 \)
\(\Rightarrow {{\left( x-\frac{7}{4} \right)}^{2}}-\frac{49}{16}+\frac{3}{2}=0 \)
\(\Rightarrow {{\left( x-\frac{7}{4} \right)}^{2}}-\left( \frac{49-24}{16} \right)=0 \)
\(\Rightarrow {{\left( x-\frac{7}{4} \right)}^{2}}-\frac{25}{16}=0 \)
\(i.e.,{{\left( x-\frac{7}{4} \right)}^{2}}=\frac{25}{16}\Rightarrow x-\frac{7}{4}=\pm \frac{5}{4} \)
\(i.e.,x-\frac{7}{4}=\frac{5}{4}\text{   or    }x-\frac{7}{4}=-\frac{5}{4}\text{  } \)
\(\Rightarrow x=\frac{7}{4}+\frac{5}{4}\text{   or    }x=\frac{7}{4}-\frac{5}{4}\text{  } \)
\(\Rightarrow x=3\text{  or    }x=\frac{1}{2} \)

Example 2:    Find the roots of the quadratic equation 4x2 + 4√3x + 3 = 0
Sol.    4x2 + 4√3x + 3 = 0
\( \Rightarrow {{x}^{2}}+\sqrt{3}x+\frac{3}{4}=0 \)
\(i.e.,\text{ }{{x}^{2}}+2\times x\times \frac{\sqrt{3}}{2}+{{\left( \frac{\sqrt{3}}{2} \right)}^{2}}-{{\left( \frac{\sqrt{3}}{2} \right)}^{2}}+\frac{3}{4}=0 \)
\(\Rightarrow {{\left( x+\frac{\sqrt{3}}{2} \right)}^{2}}-\frac{3}{4}+\frac{3}{4}=0 \)
\(i.e.,{{\left( x+\frac{\sqrt{3}}{2} \right)}^{2}}=0 \)
\(\Rightarrow x+\frac{\sqrt{3}}{2}=0\text{    and    }x=\frac{-\sqrt{3}}{2} \)

Example 3:    Find the roots of the quadratic equation 2x2 + x + 4 = 0
Sol.    2x2 + x + 4 = 0
\(\Rightarrow {{x}^{2}}+\frac{x}{2}+2=0 \)
\(i.e.,~{{x}^{2}}+2\times x\times \frac{1}{4}+{{\left( \frac{1}{4} \right)}^{2}}-{{\left( \frac{1}{4} \right)}^{2}}+2=0 \)
\(\Rightarrow {{\left( x+\frac{1}{4} \right)}^{2}}-\frac{1}{16}+2=0 \)
\(\Rightarrow {{\left( x+\frac{1}{4} \right)}^{2}}+\frac{31}{16}=0 \)
\(i.e.,{{\left( x+\frac{1}{4} \right)}^{2}}=-\frac{31}{16} \)
This is not possible as the square of a real number can not be negative.

Foe More Solved Examples