ML Aggarwal Class 9 Trigonometric Ratios and Standard Angles Chapter Test

ML Aggarwal Class 9 Solutions Chapter 18 Chapter Test

Question 1.
Find the values of :
(i) sin² 60° – cos² 45° + 3tan² 30°
(ii) \(\frac{2 \cos ^{2} 45^{\circ}+3 \tan ^{2} 30^{\circ}}{\sqrt{3} \cos 30^{\circ}+\sin 30^{\circ}}\)
(iii) sec 30° tan 60° + sin 45° cosec 45° + cos 30° cot 60°
Answer:
(i) sin² 60° – cos² 45° + 3tan² 30°

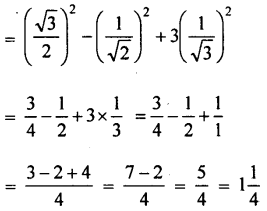
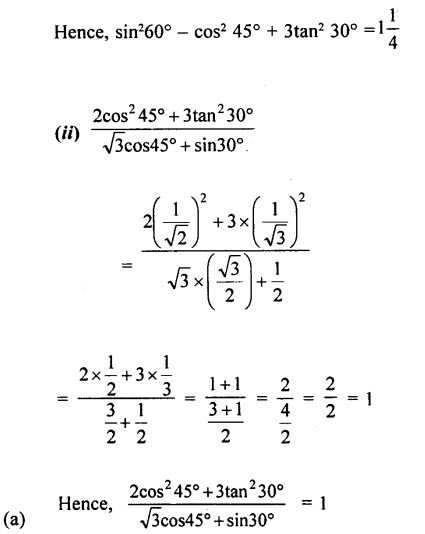
Question 2.
Taking A = 30°, verify that
(i) cos⁴ A – sin⁴ A = cos 2A
(ii) 4cos A cos (60° – A) cos (60° + A) = cos 3 A.
Answer:
(i) cos⁴ A – sin⁴ A = cos 2A
Taking A = 30°
L.H.S.: cos⁴ A – sin⁴ A = cos⁴ 30° – sin⁴ 30°

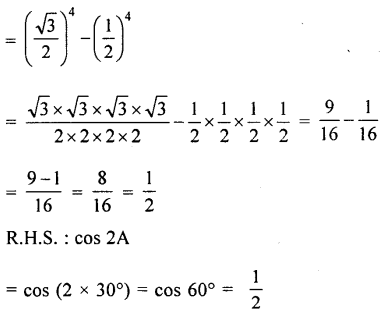
Hence, L.H.S. = R.H.S. result is proved.
(ii) 4 cos A cos (60°- A) cos (60° + A) = cos 3 A
L.H.S. = 4cos A cos (60° – A) cos (60° + A)
= 4cos 30° cos (60° – 30°) cos (60° + 30°) [∵ taking A = 30°]
= 4cos 30° cos 30° cos 90° = 4 × \(\frac{\sqrt{3}}{2} \times \frac{\sqrt{3}}{2}\) × 0 = 0
R.H.S.: cos 3A
= cos (3 × 30°) = cos 90° = 0
Hence, L.H.S. = R.H.S. result is proved.

Question 3.
If A = 45° and B = 30°, verify that

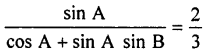
Answer:

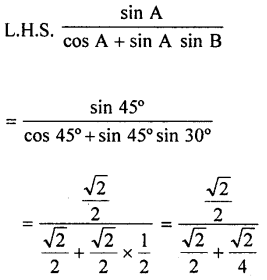
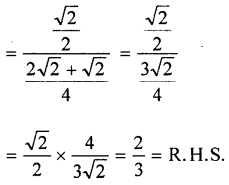
Question 4.
Taking A = 60° and B = 30°, verify that

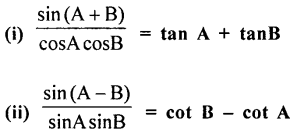
Answer:
(i) A = 60°, B = 30°

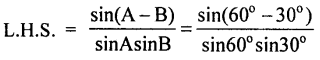
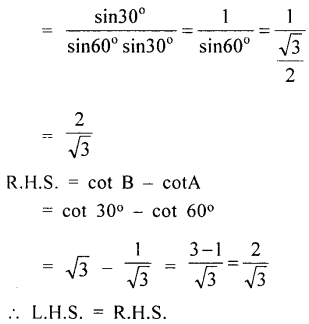
Question 5.
If \( \sqrt2 \) tan 2 θ = \( \sqrt6 \) and θ° < 2θ < 90°, find the value of sin θ + \( \sqrt3 \) cos θ – 2 tan² θ.
Answer:

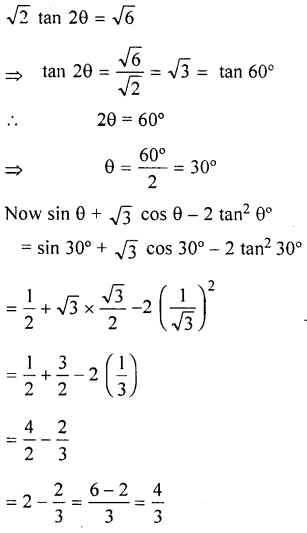
Question 6.
If 3θ is an acute angle, solve the following equations for θ :
(i) ( cosec 3θ – 2) (cot 2θ – 1) = 0

Question P.Q.
(tanθ – 1) (cosec 3θ – 1) = 0
Answer:
(i) (cosec 3θ – 2) (cot 2θ – 1) = 0
Either, cosec 3θ – 2 or cot 2θ – 1 = 0
⇒ cosec 3θ = 2 or cot 2θ = 1
⇒ cosec 3θ = cosec 3θ° or cot 2θ =cot 45°
⇒ 3θ = 30° or 2θ = 45°

(tanθ – 1) (cosec 3θ – 1) = 0
Either tanθ – 1 = 0 or cosec 3θ – 1 = 0
⇒ tanθ = 1 or cosec 3θ = 1
⇒ tanθ = tan45° or cosec 3 θ = cosec 90°
⇒ θ = 45° or 3θ = 90° or θ = \(\frac{90^{\circ}}{3}\)
= 30°
Hence, θ = 45° or 30°

Question 7.
If tan (A + B) = \( \sqrt3 \) and tan (A – B) = 1 and A, B (B < A) are acute angles, find the values of A and B.
Answer:
Given that tan (A + B) = \( \sqrt3 \)
⇒ tan ( A + B) = tan 60° [tan 60° = \( \sqrt3 \) ]
⇒ A + B = 60° ………..(1)
Also, tan (A – B) = 1
⇒ tan (A – B) = tan 45° [tan 45° = 1]
⇒ A – B = 45° …………..(2)
From equation (1) and (2), we get

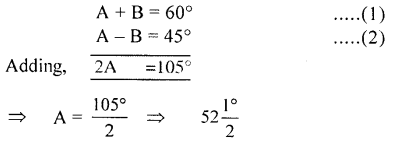
Substituting the value of A in equation (1), we get

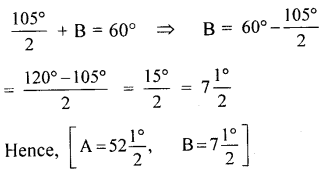
Question P.Q.
Find the values of :

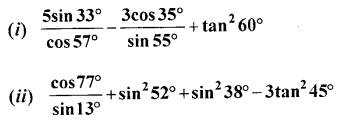
Answer:

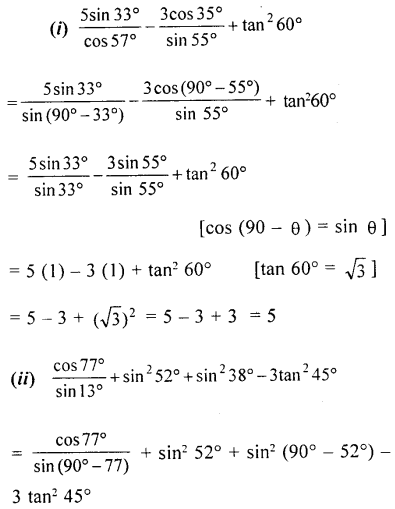
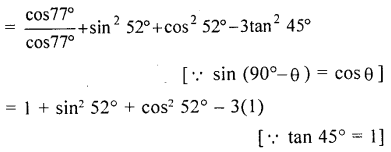
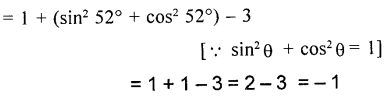
Question 8.
Without using trigonometrical tables, evaluate the following :
(i) sin² 28° + sin² 62° – tan² 45°
(ii) \(2 \frac{\cos 27^{\circ}}{\sin 63^{\circ}}+\frac{\tan 27^{\circ}}{\cot 63^{\circ}}+\cos 0^{\circ}\)
(iii) cos 18° sin 72° + sin 18° cos 72°
(iv) 5 sin 50° sec 40° – 3 cos 59° cosec 31°
Answer:
(i) sin² 28° + sin² 62° – tan² 45°
= sin² 28° + sin² (90° – 28°) – tan² 45°
= sin² 28° + cos² 28° – tan² 45°
= 1 – (1)² = 1 – 1 = 0 (∵ sin² θ + cos² θ = 1 and tan 45° = 1)

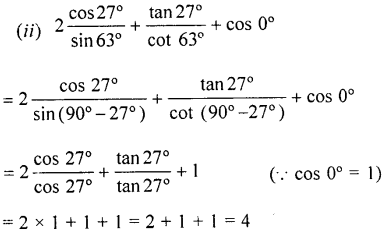
= 2 × 1 + 1 + 1 = 2 + 1 + 1= 4
(iii) cos 18° sin 12° + sin 18° cos 12°
= cos (90° – 12°) sin 72° + sin (90° – 12°) cos 12°
= sin 72°.sin 12° + cos 12° cos 12°
= sin² 12° + cos² 12° = 1 (∵ sin² θ + cos² θ = 1)
(iv) 5 sin 50° sec 40° – 3 cos 59° cosec 31°

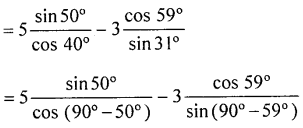
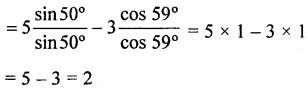
Question 9.
Prove that:

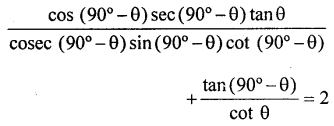
Answer:

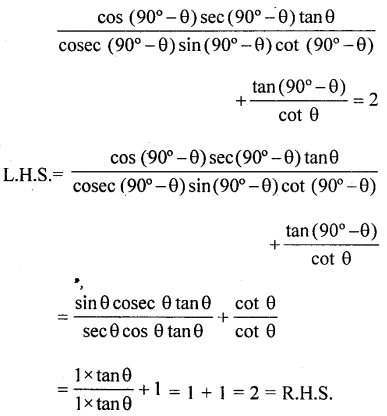
Question 10.
When 0° < A < 90°, solve the following equations :
(i) sin 3A = cos 2A
(ii) tan 5A = cotA.
Answer:
(i) sin 3A = cos 2A
⇒ sin 3A = sin (90° – 2A)
∴ 3A = 90° – 2A
3 A + 2A = 90° ⇒ 5A = 90°
∴ A = \(\frac{90^{\circ}}{5}\) = 18°
(ii) tan 5A = cot A
tan 5A = tan(90° – A)
∴ 5A = 90°- A ⇒ 5A + A = 90°
⇒ 6A = 90°
∴ A = \(\frac{90^{\circ}}{5}\) = 15°

Question 11.
Find the value of θ if
(i) sin (θ + 36°) = cos θ, where θ and θ + 36° are acute angles.
(ii) sec 4θ = cosec (θ – 20°), where 4θ and θ – 20° are acute angles.
Answer:
(i) θ and (θ + 36°) are acute angles
sin (θ + 36°) = cos θ = sin (90° – θ) {sin (90° – θ) = cos θ}
Comparing, we get
θ + 36° = 90° – θ
θ + θ = 90° – 36°
2θ = 54° ⇒ θ = \(\frac{54^{\circ}}{2}\) = 27°
∴ θ = 27°

(ii) θ and (θ – 20°) are acute angles
sec 4θ = cosec (θ – 20°)
cosec (90° – 4θ) = cosec (θ – 20°) {∵ cosec (90° – θ) = sec θ}
Comparing, we get
90° – 4θ = θ – 20°
⇒ 90° + 20° = θ + 4θ
⇒ 5θ = 110°
θ = \(\frac{110^{\circ}}{5}\) = 22°
θ = 22°

Question 12.
In the adjoining figure, ABC is right-angled triangle at B and ABD is right angled triangle at A. If BD ⊥ AC and BC = 2\( \sqrt3 \) cm, find the length of AD.

Solution:
∆ABC and ∆ABD are right angled triangles
in which \( \sqrt \) = 90° and \( \sqrt \) = 90°
BC = 2\( \sqrt3 \) cm AC and BD intersect each other at E at right angle and ∠CAb = 30°.
Now in right ∆ABC,
tan θ = \(\frac{B C}{A B}\) ⇒ tan 30°= \(\frac{2 \sqrt{3}}{\mathrm{AB}}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{2 \sqrt{3}}{\mathrm{AB}}\)
⇒ AB = 2\( \sqrt3 \) × \( \sqrt3 \) = 2 × 3 = 6 cm.
In ∆ABE, ∠EAB = 30°
and ∠EAB = 90°
∴ ∠ABE or ∠ABD = 180° – 90° – 30°
= 60°
Now in right ∆ABD,
tan 60° = \(\frac{\mathrm{AD}}{\mathrm{AB}}\) ⇒ \( \sqrt3 \) = \(\frac{\mathrm{AD}}{6}\)
⇒ AD = 6\( \sqrt3 \) cm.

ML Aggarwal Class 9 Solutions for ICSE Maths